Speed is a fundamental concept in physics that plays a crucial role in understanding motion, dynamics, and kinematics. At its core, the speed equation helps us quantify how fast an object is moving in relation to time. Whether you're a budding physicist or just curious about the world of physics, mastering the speed equation is essential. With its wide applications in various scientific and real-world scenarios, the speed equation serves as a cornerstone for numerous concepts in physics.

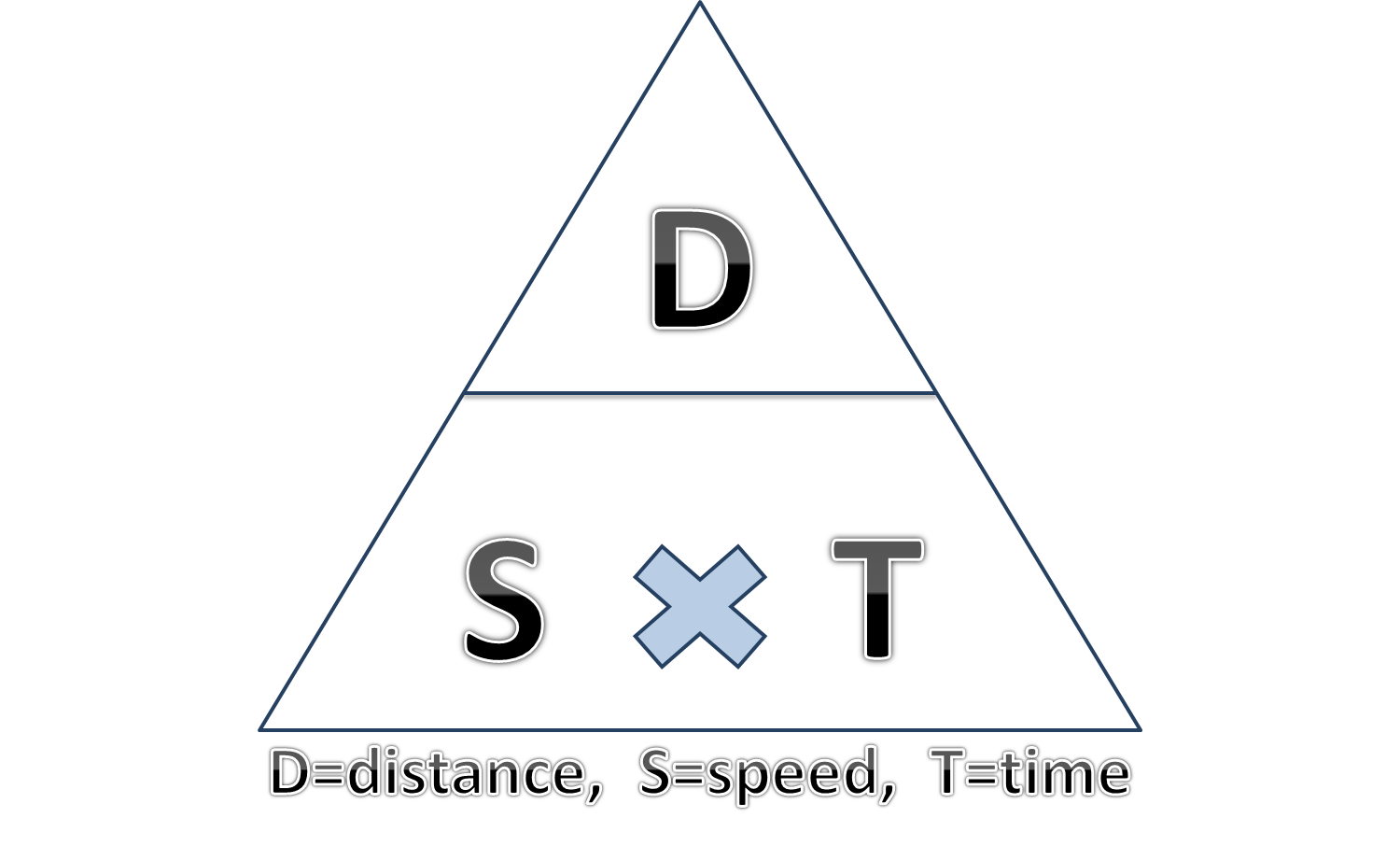
In physics, the speed of an object is defined as the distance it covers over a specified period. The speed equation is a simple yet powerful tool: Speed = Distance / Time. This equation allows scientists and researchers to calculate the rate of movement, providing insights into the behavior of moving objects. Understanding this equation is not only vital for academic purposes but also for practical applications such as transportation, engineering, and technology.

As we delve deeper into this guide, we will explore the intricacies of the speed equation physics, its real-world applications, and how it intertwines with other key concepts in science. With a focus on clarity and engagement, this article aims to provide a comprehensive overview of the speed equation, ensuring that readers of all levels can grasp its significance and utility. Let's embark on this informative journey to unravel the mysteries of speed in the realm of physics.

What is Speed in Physics?
Why is Understanding Speed Important?
Deriving the Speed Equation
Applications of Speed Equation in Real Life
Are There Limitations to the Speed Equation?
How Does Speed Relate to Velocity and Acceleration?
Historical Context of Speed Equation Physics
Units of Measurement for Speed
Experiment Examples Using Speed Equation
Common Misconceptions About Speed Equation Physics
Can Speed Equation Explain Relativity?
Tips for Calculating Speed Accurately
Learning Resources for Speed Equation Physics
Future Implications of Speed Equation in Physics
Frequently Asked Questions
Conclusion

What is Speed in Physics?

Speed, in physics, is the measure of how quickly an object moves from one place to another. It is a scalar quantity, meaning it only has magnitude and no direction. The simplicity of the concept might be misleading because speed is a fundamental part of various physics topics, including motion, energy, and dynamics. Understanding speed is crucial for students, scientists, and engineers alike as it forms the basis for more complex theories and applications.

Why is Understanding Speed Important?

Understanding speed is critical for several reasons:

It helps in predicting and analyzing motion.
It is essential for calculating travel time and optimizing routes.
Speed plays a role in safety measures, especially in transportation.
It is used in designing machines and vehicles for efficiency.

Grasping the concept of speed can lead to innovations and improvements in various fields such as engineering, aeronautics, and even everyday technology.

Deriving the Speed Equation

The speed equation is derived from the basic definition of speed itself. Given that speed is the distance traveled per unit of time, the formula can be expressed as:

Speed = Distance / Time

Where:

Speed is measured in units such as meters per second (m/s) or kilometers per hour (km/h).
Distance is the total path covered, usually measured in meters or kilometers.
Time is the duration taken to cover the distance, typically measured in seconds or hours.

This equation is pivotal for calculations involving motion, allowing us to determine how fast an object is moving under various conditions.

Applications of Speed Equation in Real Life

The speed equation is applied in numerous real-world scenarios, making it one of the most utilized formulas in physics and everyday life. Some notable applications include:

Transportation: Calculating travel time and fuel efficiency for vehicles.
Sports: Analyzing athlete performance by measuring speed and endurance.
Astronomy: Estimating the speed of celestial bodies to understand their trajectories.
Engineering: Designing machines and systems that require precise speed measurements.

The versatility and simplicity of the speed equation make it an indispensable tool for various disciplines.

Are There Limitations to the Speed Equation?

While the speed equation is a powerful tool, it does have its limitations:

It does not account for direction, which is crucial for vector quantities.
It assumes constant speed without considering acceleration or deceleration.
In real-world scenarios, factors like friction and air resistance can affect speed calculations.

Despite these limitations, the speed equation remains valuable for its simplicity and ease of use in basic calculations.

Speed, velocity, and acceleration are related but distinct concepts in physics:

Speed is a scalar quantity that measures how fast an object is moving.
Velocity includes both the speed and direction of an object's motion, making it a vector quantity.
Acceleration refers to the rate of change of velocity over time, indicating how quickly an object speeds up or slows down.

These concepts are interconnected, with speed providing a foundation for understanding more complex phenomena in motion and dynamics.

Historical Context of Speed Equation Physics

The study of speed and motion dates back to ancient times, with notable contributions from figures such as Galileo and Newton. Galileo's experiments on motion laid the groundwork for understanding constant speed, while Newton's laws of motion further expanded the concepts of speed, velocity, and acceleration.

Over time, the speed equation has evolved, becoming a fundamental part of physics curricula worldwide. Its simplicity and applicability have ensured its continued relevance in both academic and practical settings.

Units of Measurement for Speed

Speed is measured using various units, depending on the context:

Meters per second (m/s): The standard unit in the International System of Units (SI), commonly used in scientific contexts.
Kilometers per hour (km/h): Widely used in transportation and everyday contexts.
Miles per hour (mph): Commonly used in countries like the United States and the United Kingdom.

The choice of unit depends on the scale and purpose of the measurement, ensuring precision and clarity.

Experiment Examples Using Speed Equation

Conducting experiments using the speed equation can enhance understanding and provide practical insights:

Measuring the speed of a rolling ball: By measuring the distance a ball travels and the time taken, students can calculate its speed.
Speed of a car on a track: Using sensors and timers, one can determine the speed of a car at various points along a track.
Astronomical observations: Calculating the speed of celestial bodies by measuring their displacement over time.

These experiments provide hands-on experience with the speed equation, reinforcing its principles and applications.

Common Misconceptions About Speed Equation Physics

Despite its simplicity, the speed equation is often misunderstood, leading to misconceptions such as:

Confusing speed with velocity, which includes direction.
Assuming speed is always constant, ignoring acceleration.
Overlooking factors like friction and resistance that can affect speed.

Clarifying these misconceptions is crucial for a proper understanding of motion and dynamics.

Can Speed Equation Explain Relativity?

The speed equation is a fundamental concept in classical physics but has limitations in the context of relativity. Einstein's theory of relativity introduced new perspectives on motion and speed, particularly at speeds approaching the speed of light. In such scenarios, the speed equation's simplicity is insufficient, and relativistic equations are required to accurately describe motion.

Nevertheless, the speed equation remains a valuable tool for everyday scenarios and classical mechanics, where relativistic effects are negligible.

Tips for Calculating Speed Accurately

To ensure accurate speed calculations, consider the following tips:

Use precise measurements for distance and time.
Account for factors like friction and air resistance, if applicable.
Ensure consistent units for all measurements.
Double-check calculations for errors or discrepancies.

These tips can enhance the accuracy and reliability of speed calculations, whether in experiments or real-world applications.

Learning Resources for Speed Equation Physics

Numerous resources are available for those interested in learning more about speed equation physics:

Textbooks: Many physics textbooks cover speed and motion extensively, providing theoretical and practical insights.
Online Courses: Platforms like Coursera and Khan Academy offer courses on physics, including speed and motion.
Interactive Simulations: Websites like PhET provide simulations that allow users to explore speed and motion concepts interactively.

These resources can enhance understanding and provide diverse perspectives on speed equation physics.

Future Implications of Speed Equation in Physics

The speed equation will continue to play a significant role in physics and beyond. As technology advances, new applications and interpretations of speed may emerge, influencing fields such as:

Transportation: Innovations in transportation technologies may rely on precise speed calculations for optimization and safety.
Astronomy: Future astronomical discoveries may depend on understanding the speed of celestial bodies and their interactions.
Engineering: As engineering techniques evolve, the need for accurate speed measurements will remain crucial for efficiency and design.

The speed equation's simplicity and versatility ensure its continued relevance and potential for future advancements.

Frequently Asked Questions

What is the basic speed formula in physics? The basic speed formula is Speed = Distance / Time.
How does speed differ from velocity? Speed is a scalar quantity with only magnitude, while velocity is a vector quantity that includes direction.
Why is speed important in daily life? Speed is important for predicting motion, optimizing travel, and ensuring safety in various contexts.
Can speed be negative? Speed, being a scalar, cannot be negative. However, velocity can be negative if it indicates a direction opposite to the reference.
What units are commonly used to measure speed? Common units include meters per second (m/s), kilometers per hour (km/h), and miles per hour (mph).
Is the speed equation applicable in all motion scenarios? The speed equation is applicable in most scenarios, but not when relativistic effects are significant, as in the case of speeds approaching the speed of light.

Conclusion

The speed equation is a fundamental aspect of physics, providing a simple yet powerful tool for understanding motion and dynamics. Its applications span various fields, from transportation to astronomy, making it indispensable for scientific and practical purposes. While it has limitations, particularly in the context of relativity, its utility in everyday scenarios and classical mechanics remains unparalleled. By mastering the speed equation, individuals can gain deeper insights into the principles of motion and their implications in the real world.