Mastering Pythagorean Theorem Hard Problems For Success

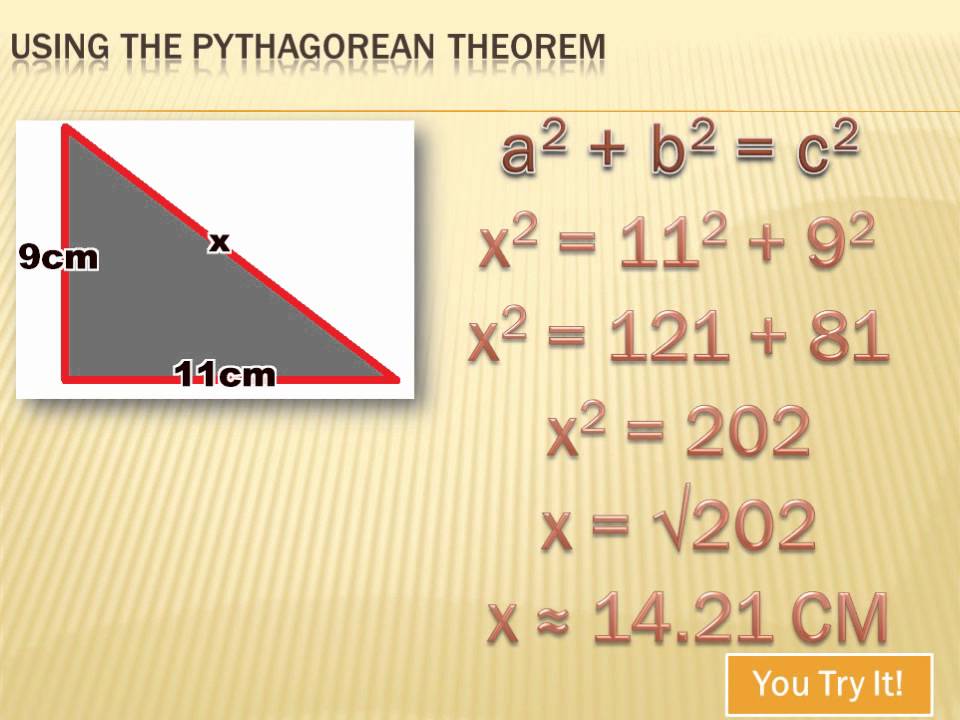
The Pythagorean Theorem is more than just a simple equation; it's a fundamental principle that has fascinated mathematicians for centuries. It serves as a cornerstone in geometry, helping us understand the relationships between the sides of right-angled triangles. However, solving Pythagorean theorem problems can sometimes present challenges, especially when faced with complex scenarios that require deep analytical skills.

When diving into Pythagorean theorem hard problems, students often encounter a range of difficulties. These can include multi-step solutions, the integration of additional geometric concepts, and sometimes even algebraic manipulations. Despite these challenges, mastering these problems is rewarding, as it not only enhances mathematical understanding but also hones critical thinking and problem-solving abilities. With the right approach and strategies, tackling these problems can become an engaging and insightful experience.

In this comprehensive guide, we aim to equip you with the necessary tools and methods to conquer Pythagorean theorem hard problems. From understanding the theorem's origins to detailed problem-solving techniques, each section is designed to build your confidence and proficiency. Whether you are a student looking to excel in math or a teacher seeking to provide enriched learning experiences, this article will serve as a valuable resource in your educational journey.

Table of Contents

• Introduction to the Pythagorean Theorem
• What is the History of the Pythagorean Theorem?
• Understanding the Pythagorean Theorem
• Real-Life Applications of the Pythagorean Theorem
• How Can We Tackle Complex Problems?
• Integrating Geometry Concepts
• Using Algebra in Pythagorean Problems
• Strategies for Solving Hard Problems
• Examples of Pythagorean Theorem Hard Problems
• Why is Practice Essential?
• Enhancing Critical Thinking Skills
• Educational Resources and Tools
• What Are Common Mistakes to Avoid?
• FAQs on Pythagorean Theorem Hard Problems
• Conclusion

Introduction to the Pythagorean Theorem

The Pythagorean Theorem is a fundamental concept in mathematics that describes the relationship between the sides of a right-angled triangle. The theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.

Mathematically, the theorem can be expressed as a² + b² = c², where c represents the length of the hypotenuse, and a and b represent the lengths of the triangle's other two sides. This equation forms the basis for many geometric calculations and is essential in various fields such as architecture, engineering, and physics.

Despite its simplicity, the Pythagorean Theorem has profound implications and is used in numerous mathematical problems, including those that appear complex at first glance. Understanding its principles is crucial for solving advanced mathematical problems, making it a vital tool in a student's mathematical toolkit.

What is the History of the Pythagorean Theorem?

Named after the ancient Greek mathematician Pythagoras, the Pythagorean Theorem has a rich history that dates back to approximately 500 BC. Despite being attributed to Pythagoras, evidence suggests that the theorem was known to the Babylonians and Indians centuries before Pythagoras' time. However, it's Pythagoras who is credited with the first formal proof of the theorem.

The theorem became a cornerstone of Greek mathematics and was pivotal in the development of Euclidean geometry. Over time, mathematicians across different cultures and eras have contributed to the understanding and application of the theorem, expanding its reach and significance.

The Pythagorean Theorem's influence extends beyond mathematics into philosophy and science, symbolizing the unity of theory and practice. Its historical journey underscores the timelessness of mathematical principles and the continuous quest for knowledge and understanding.

Understanding the Pythagorean Theorem

At its core, the Pythagorean Theorem is about understanding the spatial relationships between the sides of a right-angled triangle. The theorem can be visualized by considering a right triangle where the longest side, known as the hypotenuse, and the other two sides form the right angle.

To fully grasp the theorem, it's essential to explore its geometric interpretation. Imagine a square constructed on each side of a right triangle. The area of the square on the hypotenuse will be equal to the sum of the areas of the squares on the other two sides. This visualization helps in comprehending the theorem's essence and its application in various geometric constructions.

Further, the Pythagorean Theorem is not limited to right triangles alone. It also plays a crucial role in coordinate geometry, allowing the calculation of distances between two points on a plane. This versatility makes it an invaluable tool in both theoretical and practical mathematics.

Real-Life Applications of the Pythagorean Theorem

The Pythagorean Theorem's utility extends beyond classroom exercises, finding applications in numerous real-world scenarios. In architecture and construction, it helps in determining the appropriate lengths of materials needed to create right angles, ensuring structural integrity.

In navigation and mapping, the theorem aids in calculating the shortest path between two points, a principle applied in GPS technology. Similarly, in physics, the theorem assists in resolving forces and understanding wave patterns, highlighting its interdisciplinary significance.

Furthermore, the Pythagorean Theorem plays a role in computer graphics, where it helps in rendering images with accurate dimensions and perspectives. These applications underscore the theorem's practicality and its enduring relevance in modern technology and science.

How Can We Tackle Complex Problems?

When faced with complex Pythagorean theorem problems, it's important to approach them systematically. Start by carefully analyzing the problem statement and identifying the known and unknown variables. Visualizing the problem using diagrams or sketches can provide clarity and insight.

Consider breaking down the problem into smaller, more manageable parts. This approach allows for the application of different mathematical principles, such as algebraic manipulation or trigonometric identities, to find a solution. It's also helpful to explore alternative methods or perspectives, as some problems may have multiple solutions.

Additionally, practice is key to mastering complex problems. Regularly engaging with challenging exercises and seeking feedback from teachers or peers can improve problem-solving skills and build confidence.

Integrating Geometry Concepts

The Pythagorean Theorem is closely linked with other geometric concepts, which can be integrated to solve complex problems. Understanding properties of similar triangles, for example, can provide additional insights and simplify problem-solving.

In some cases, the use of geometric transformations, such as rotations or reflections, can offer new perspectives on a problem. These transformations can help in visualizing relationships between different elements of a geometric figure, leading to more efficient solutions.

Moreover, the integration of concepts like congruence and symmetry can enhance the understanding of Pythagorean theorem problems, allowing for a more comprehensive approach to geometry.

Using Algebra in Pythagorean Problems

Algebraic manipulation is often necessary when solving Pythagorean theorem hard problems. The ability to rearrange equations, factor expressions, and simplify terms is essential for finding solutions.

Incorporating algebraic techniques, such as solving systems of equations or using quadratic formulas, can help in tackling more complex problems. These techniques allow for the exploration of different solution paths and the identification of potential pitfalls.

Furthermore, algebra can be used to generalize solutions and create formulas that apply to a broader range of problems, showcasing the interconnectedness of mathematical concepts.

Strategies for Solving Hard Problems

Developing effective strategies is crucial for solving Pythagorean theorem hard problems. One such strategy is the use of problem-solving frameworks, which provide a structured approach to tackling complex questions.

Another strategy involves the application of estimation techniques to verify the reasonableness of a solution. This can help in identifying errors and ensuring accuracy in calculations.

Additionally, leveraging technology, such as graphing calculators or computer software, can assist in visualizing problems and exploring different solution paths, offering valuable support in the problem-solving process.

Examples of Pythagorean Theorem Hard Problems

To illustrate the application of the Pythagorean Theorem, let's consider some challenging problems:

• Problem 1: A ladder leans against a wall, forming a right triangle with the ground. If the ladder is 13 meters long and the distance from the base of the ladder to the wall is 5 meters, how high is the top of the ladder on the wall?
• Problem 2: In a rectangular park, a diagonal path is constructed from one corner to the opposite corner. If the length of the park is 30 meters and the width is 40 meters, what is the length of the diagonal path?
• Problem 3: A square and a triangle share a side. If the side of the square measures 10 units and the hypotenuse of the triangle is 15 units, find the length of the other side of the triangle.

Solutions to these problems require understanding the properties of right triangles and applying the Pythagorean Theorem strategically. Each problem presents unique challenges that test mathematical reasoning and creativity.

Why is Practice Essential?

Consistent practice is essential for mastering Pythagorean theorem hard problems. Regular engagement with a variety of questions enhances familiarity with different problem types and builds confidence in applying mathematical principles.

Practice also helps in identifying common pitfalls and misconceptions, allowing for the development of more accurate problem-solving techniques. It provides opportunities to experiment with different strategies and approaches, fostering a deeper understanding of the theorem.

Moreover, practice instills a sense of discipline and perseverance, essential qualities for success in mathematics and beyond.

Enhancing Critical Thinking Skills

Solving Pythagorean theorem hard problems can significantly enhance critical thinking skills. These problems require careful analysis, logical reasoning, and the ability to make connections between different mathematical concepts.

Engaging with challenging problems encourages the development of a systematic approach to problem-solving, promoting the use of evidence-based reasoning and decision-making.

Furthermore, the exploration of multiple solution paths and the evaluation of their effectiveness foster a flexible mindset, essential for tackling complex challenges in various fields.

Educational Resources and Tools

Numerous educational resources and tools are available to support the learning of Pythagorean theorem hard problems. Online platforms, such as Khan Academy and Coursera, offer comprehensive courses and tutorials on geometry and algebra.

Interactive tools, such as GeoGebra, provide opportunities for hands-on exploration and visualization of geometric concepts, aiding in the understanding of the theorem.

Additionally, textbooks and workbooks designed for different learning levels offer structured exercises and problem sets, allowing for targeted practice and skill development.

What Are Common Mistakes to Avoid?

When solving Pythagorean theorem hard problems, several common mistakes can hinder progress. One such mistake is incorrect identification of the hypotenuse, leading to errors in calculations.

Another common error is the misapplication of algebraic techniques, resulting in incorrect simplification or factorization of expressions. It's important to carefully follow each step and verify calculations to avoid these pitfalls.

Additionally, overlooking key details in the problem statement can lead to incorrect assumptions and solutions. Thoroughly understanding the problem and its requirements is crucial for achieving accurate results.

FAQs on Pythagorean Theorem Hard Problems

Q1: What is the Pythagorean Theorem?

The Pythagorean Theorem is a mathematical principle that states that in a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.

Q2: How is the Pythagorean Theorem used in real life?

The Pythagorean Theorem is used in various real-life applications, including construction, navigation, and physics, to calculate distances and ensure accuracy in measurements.

Q3: What are some common mistakes when solving Pythagorean theorem problems?

Common mistakes include incorrect identification of the hypotenuse, misapplication of algebraic techniques, and overlooking details in the problem statement.

Q4: How can I improve my skills in solving Pythagorean theorem hard problems?

Improving skills requires consistent practice, understanding of geometric and algebraic concepts, and the application of effective problem-solving strategies.

Q5: What resources are available for learning Pythagorean theorem problems?

Resources include online platforms like Khan Academy, interactive tools like GeoGebra, and textbooks with structured exercises.

Q6: Why is the Pythagorean Theorem important in mathematics?

The Pythagorean Theorem is important because it provides a foundation for understanding geometric relationships and is widely used in various mathematical and scientific applications.

Conclusion

Mastering Pythagorean theorem hard problems is a rewarding endeavor that enhances mathematical understanding and problem-solving skills. By exploring the theorem's history, applications, and problem-solving strategies, learners can build a solid foundation for tackling complex challenges. With consistent practice and the use of educational resources, anyone can develop the proficiency needed to excel in mathematics and beyond. The Pythagorean Theorem remains a timeless principle, bridging the gap between theory and practice, and inspiring generations of mathematicians and scholars.